Vibration of molecules

Introduction

From the pictures in the books of chemistry one can get the impression that molecules are static entities. On the contrary, molecules are very active and dynamic. When they are not dancing they are exercising.

Atoms in molecules are held together by chemical bonds. These bonds are not rigid, but elastic like tiny springs. When let alone the length of the bonds reaches the equilibrium bond distance. But a finite temperature or any other perturbation like a collision or a photon of light shakes the atoms and the bond length start oscillating around the equilibrium bond distance. The speed at which the bonds oscillate are characteristic of each molecule. As a delicate musical instruments each molecule has its characteristic frequencies. The frequencies of vibration of molecules depend on the atoms and the chemical bonds that bind them. Heavy atoms with soft bonds will oscillate slowly, but light atoms with hard bonds will oscillate at high frequency.

We spectroscopists tend to think of molecules in terms of their music, which makes them even more beautiful. We look carefully for resonances until we find their frequencies and we finally extract all their secrets. In that way we found the composition of substances in the lab and in far distant worlds.

To see the importance of molecular vibrations you can have a look at the excellent page Water Absorption Spectrum written by Martin Chaplin.

Diatomic molecules

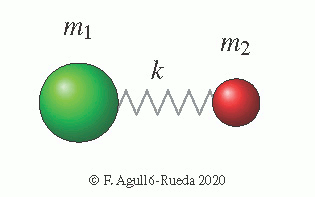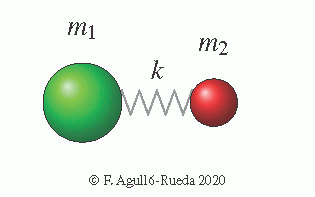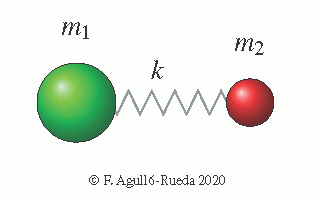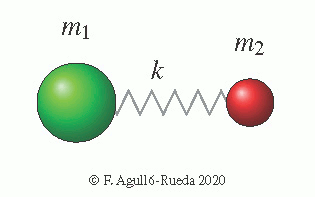
The simplest case is that of diatomic molecules, formed by only two atoms joined by a bond. The only possible mode of vibration is the bond stretching, where both atoms alternatively get closer or separate.

Examples: $\ce{H2}$ ($\ce{H-H}$), $\ce{HCl}$ ($\ce{H-Cl}$), $\ce{O2}$ ($\ce{O=O}$), $\ce{N2}$ ($\ce{N#N}$)

The force constant of a bond

The force constant $k$ measures the rigidity of a bond. Its exact value is difficult to calculate because it depends on the electronic structure of the bond or how the electrons orbit around the atomic nuclei of the molecule. This involves the use of quantum mechanics and is very sensitive to the environment. But approximate values for common bonds in simple molecules and organic molecules are tabulated. Also, as a general trend for a bond between two specific atoms, the magnitude of $k$ increases following the sequence single, double and triple bond. Also, a shortening of the equilibrium bond length will generally increase the value of $k$.

The reduced mass

For a diatomic molecule consisting of two atoms of masses $m_1$ and $m_2$, the reduced mass is defined as:

$$\mu = \frac{m_1 m_2}{m_1 + m_2}$$

This defined as the mass of an atom bonded to an infinite mass that oscillates with the same frequency when the force constant is also $k$.

The mass depends on the chemical element and also on the particular isotope. It depends therefore on the chemical composition of the molecule. For a diatomic molecule with two identical atoms of mass $m$ the reduced mass is one half of that mass, $\mu = m/2$.

The exact value of the masses can be readily obtained from any periodic table of the chemical elements. It is the easy part of calculating the frequency.

Frequency of vibration

A a diatomic molecule behaves as an harmonic oscillator, a model system in physics where a mass is kept around a fixed equilibrium position by restoring forces. These forces become stronger when the mass moves further away from that position. In an harmonic oscillator the force is elastic, that is, proportional to the deviation. This is an ideal case, but for small deviations, many oscillating systems can be cosidered an harmonic oscillator. That applies to a mass attached to a spring, to a pendulum, to a swing, to a rubber band, and also to molecules.

As in any harmonic oscillator the vibrational frequency of a diatomic molecules is:

$$\nu = \frac{1}{2\pi} \sqrt{\frac{ k }{ \mu }},$$

where $k$ is the force constant and $\mu$ is the reduced mass.

As the mass is in the denominator, lighter atoms will produce a larger frequency. That is why hydrogen atoms vibrate at the highest observed frequencies, above 3000 cm$^{-1}$. The force constant is in the numerator, therefore the frequency of vibration of triple bonds is higher than for the corresponding double and single bonds.

Polyatomic molecules

Molecule with $n$ atoms.

$3 n - 6$ for nonlinear molecules.

Examples.

group theory